Negative Convexity is a term used in fixed-income investing, referring to the shape of a bond's yield curve and how it reacts to interest rate changes. In a negative convexity scenario, as interest rates decrease, the price appreciation of a bond is less than the price depreciation for a corresponding increase in rates. Conversely, as rates rise, the price decline is more pronounced than the price appreciation for a comparable decrease in rates. This characteristic is most commonly seen in callable bonds, where the issuer has the right to repay the bond before maturity when interest rates fall. The concept of negative convexity is essential for investors as it could affect the total return and risk profile of their bond portfolio. Understanding this principle aids in making informed decisions in a fluctuating interest rate environment, ensuring optimal investment strategy planning and risk management. When interest rates decrease, bond prices generally increase. However, the price of a bond with negative convexity does not rise as much as it would if it had positive convexity. This phenomenon occurs due to the issuer's potential to call the bond, limiting the price increase. Prepayment risk, especially pertinent in the Mortgage-Backed Securities (MBS) market, is the risk that a borrower will repay a loan or other borrowed funds before the maturity date. This risk increases the potential for negative convexity as prepayment can occur when interest rates are falling, limiting price appreciation. Negative convexity generally results in lower bond prices compared to bonds of similar maturities, durations, and yields that do not have the feature of negative convexity. This pricing discrepancy is due to the additional risk that negative convexity imposes on the bondholder. Callable bonds exhibit negative convexity because the issuer has the right, but not the obligation, to call the bond before its maturity date. This means that when interest rates fall, the issuer can buy back the bonds, which limits the price appreciation that the bondholder would otherwise experience. Negative convexity is a significant issue in MBS due to prepayment risk. Homeowners typically refinance their mortgages when interest rates fall, resulting in a prepayment of the original loan. This scenario is similar to the issuer of a bond calling it back, limiting the price appreciation of the MBS. Fixed-income securities, such as bonds and MBS, are susceptible to interest rate risk. The effects of this risk are more pronounced when the securities exhibit negative convexity, making understanding this concept crucial for fixed-income investors. Duration measures the sensitivity of a bond's price to changes in interest rates. Convexity complements duration by measuring the rate of change of the bond's duration. Negative convexity implies that as interest rates decrease, the duration of the bond decreases, leading to smaller price increases. There are various software and financial calculators available to investors to measure convexity. These tools include offerings from popular financial services companies, such as Bloomberg terminals and Morningstar Direct, which provide advanced fixed-income analytics. The convexity adjustment formula quantifies the effect of convexity on a bond's price. This formula is useful for pricing callable bonds and MBS, as these instruments often exhibit negative convexity. Investors should be aware of the risks associated with negative convexity, especially if they hold callable bonds or MBS. Effective risk management strategies should consider the potential impact of negative convexity on bond prices. The shape of the yield curve can affect the degree of negative convexity. If the yield curve is steep, then the impact of negative convexity on a bond's price could be significant. Negative convexity can influence the performance of a bond portfolio. Portfolios with a high degree of negative convexity could underperform when interest rates change, particularly when rates fall. Active management involves making specific investments with the goal of outperforming an investment benchmark index. Active managers could potentially limit the impact of negative convexity by selling bonds that exhibit this property. Investors can diversify their bond portfolios by including bonds with different characteristics, such as varying maturities, credit qualities, and degrees of convexity. This strategy can help mitigate the potential adverse effects of negative convexity. Derivatives such as interest rate swaps and options can be used to manage the interest rate risk associated with negative convexity. These financial instruments can help mitigate potential losses if interest rates change unfavorably. For positively convex bonds, the price increases at an accelerated rate when interest rates decrease. Conversely, when rates rise, the bond price decreases, but at a slower pace. However, negatively convex bonds react differently. Their prices increase at a slower rate when interest rates decrease, and their prices decrease at an accelerated rate when the rates rise. Positive convexity offers more favorable risk-reward dynamics for bondholders. As interest rates decrease, the bondholder gets the benefit of a faster increase in bond prices. However, with negatively convex bonds, bondholders take on higher risk. The bond price decreases more rapidly when interest rates rise, which could lead to higher losses. The positive convexity characteristic generally makes a bond more attractive to investors. This is because the bondholder has the potential to gain more when interest rates fall and lose less when rates rise. On the other hand, bonds with negative convexity are typically less appealing to investors due to the increased risk of faster depreciation in bond prices with rising interest rates. Central banks' monetary policy decisions can have a significant impact on negative convexity. If a central bank decides to raise interest rates, bonds with negative convexity could see their prices decrease rapidly. Future financial regulations could also influence the prevalence of negative convexity in the bond market. For example, regulations that limit the ability of issuers to call their bonds could reduce the instances of negative convexity. Understanding negative convexity will continue to be essential for bond investors. Those who can successfully navigate the challenges posed by negative convexity could potentially enhance their returns and reduce risk in their bond portfolios. Negative convexity, a crucial consideration in fixed-income investing, characterizes a bond's response to changing interest rates. It particularly impacts callable bonds and mortgage-backed securities due to the risk of the issuer or borrower cutting short the bond or loan life, respectively. This feature can lead to less price appreciation when interest rates fall and more pronounced price depreciation when rates rise. Through financial tools and the Convexity Adjustment Formula, investors can measure this effect on bond prices. To mitigate negative convexity, strategies like active portfolio management, diversification, and the use of derivatives are advisable. Future monetary policies, financial regulations, and the shape of the yield curve could potentially impact the prevalence and consequences of negative convexity. Thus, understanding this concept is vital for successful risk management and optimization of bond portfolio performance.Definition of Negative Convexity
Understanding the Concept of Negative Convexity
Impact of Interest Rates
Understanding Prepayment Risk
Effect of Negative Convexity on Bond Prices
Factors Leading to Negative Convexity
Callable Bonds and Negative Convexity
Mortgage-Backed Securities (MBS) and Negative Convexity
Role of Fixed Income Securities

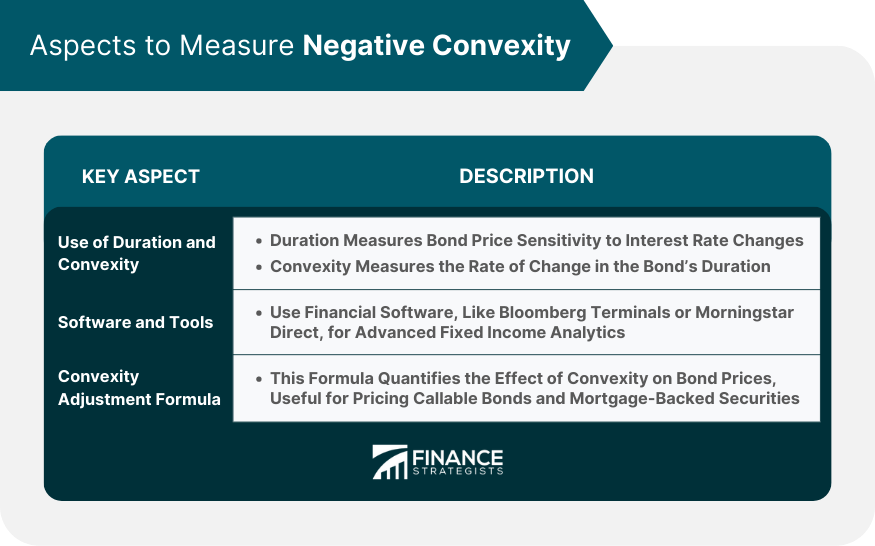
How to Measure Negative Convexity
Use of Duration and Convexity
Software and Tools for Measuring Convexity
Understanding the Convexity Adjustment Formula
Implications of Negative Convexity for Investors
Risk Management and Negative Convexity
Yield Curve Considerations
Portfolio Implications
Strategies to Mitigate the Impact of Negative Convexity
Active Portfolio Management
Diversification Strategies
Use of Derivatives
Positive Convexity vs Negative Convexity
Impact on Bond Prices With Interest Rate Changes
Risk and Reward Dynamics
Investment Attractiveness

Future of Negative Convexity
Role of Central Banks
Impact of Financial Regulations
Prospects for Investors and Negative Convexity in the Future
Final Thoughts
Negative Convexity FAQs
Negative convexity is a concept in bond investing that refers to a situation where as interest rates decrease, bond prices increase at a decreasing rate, and as interest rates increase, bond prices decrease at an increasing rate. This property is typically seen in callable bonds and mortgage-backed securities.
Negative convexity significantly impacts MBS due to the risk of prepayment. When interest rates fall, homeowners are likely to refinance their mortgages, resulting in prepayment of the original loan. This is akin to a bond being called back by the issuer, thereby limiting the price appreciation of the MBS.
Some strategies to mitigate negative convexity include active portfolio management, diversification of bond portfolios, and the use of derivatives such as interest rate swaps and options. These can help manage the interest rate risk associated with negative convexity.
A notable historical example is the 1994 bond market massacre when the Fed unexpectedly raised interest rates, causing bond prices, particularly callable bonds, and MBS, to fall dramatically. Another instance is during the 2008 financial crisis, where widespread mortgage refinancing led many mortgage-backed securities to exhibit negative convexity.
Future developments, such as monetary policy decisions by central banks and financial regulations, could influence the prevalence of negative convexity. For instance, if central banks raise interest rates, bonds with negative convexity could see their prices decrease rapidly. Similarly, regulations limiting the ability of issuers to call their bonds could reduce instances of negative convexity.
True Tamplin is a published author, public speaker, CEO of UpDigital, and founder of Finance Strategists.
True is a Certified Educator in Personal Finance (CEPF®), author of The Handy Financial Ratios Guide, a member of the Society for Advancing Business Editing and Writing, contributes to his financial education site, Finance Strategists, and has spoken to various financial communities such as the CFA Institute, as well as university students like his Alma mater, Biola University, where he received a bachelor of science in business and data analytics.
To learn more about True, visit his personal website or view his author profiles on Amazon, Nasdaq and Forbes.