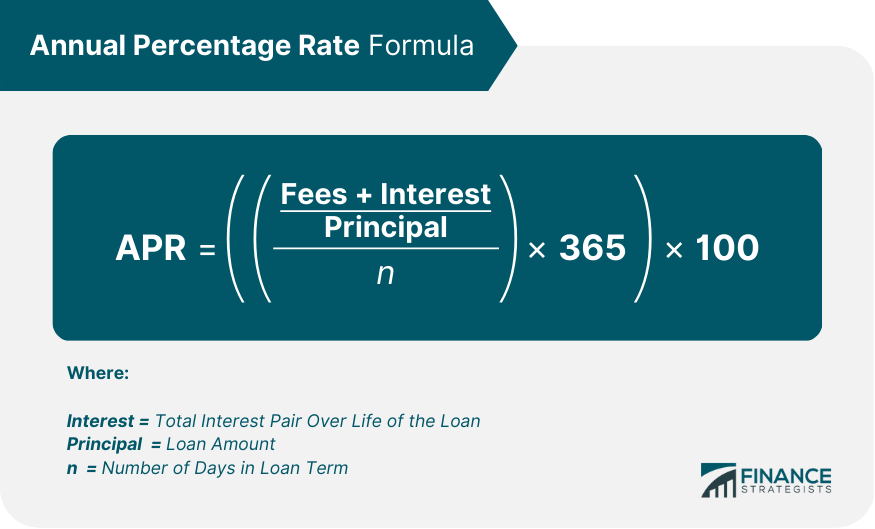
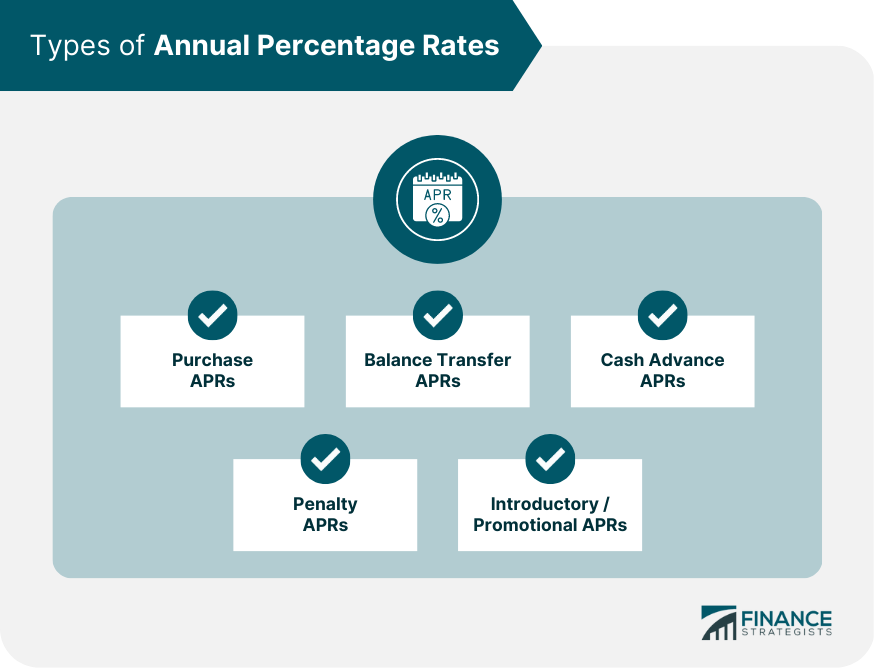
Annual Percentage Rate (APR) is the rate of interest charged on borrowing or earned through investing, expressed as a yearly rate. It is typically used to compare different types of financial products, such as credit cards, loans, and mortgages. APR is calculated by taking into account additional costs such as processing or closing fees in addition to the interest rate, and is typically higher than the advertised interest rate. For example, if you borrow money for a loan with an interest rate of 8%, your actual APR could be 10% after considering additional costs. The APR is essential to personal finance, representing the yearly rate charged for a loan or earned by an investment. Financial institutions must furnish this information in a standardized format to protect consumers from making uninformed decisions based on questionable advertising claims. The APR is only sometimes an accurate representation of the cost of borrowing, as lenders have some flexibility in calculating APR figures, omitting certain fees and costs. It is essential to note the difference between APR and Annual Percentage Yield (APY) - while both describe the annual interest rate incurred by an investment or grantee, respectively, APY takes compounding into account. APR is an annual interest rate that encapsulates the cost of borrowing or investing, taking into account not just the advertised interest rate but also monthly payments and other associated costs. The Truth in Lending Act of 1968 requires lenders to disclose their APR to borrowers before signing any agreements. Credit card companies can advertise interest rates monthly, but they must provide customers with accurate information about the APR before any agreement is finalized. The formula for APR is: For example, if you borrowed $8,000 from a bank with an interest rate of 5%, a two-year term, and an origination fee of $75, then the total cost for your loan would be $800 in interest payments. To calculate the APR, add the origination fees, and total interest paid: $75 + $800 = $875. Subsequently, divide this result by the principal amount of the loan ($875 divided by $8,000). This results in 0.1094, which you must divide again by 730 days for how long your loan lasts (0.1094 / 730). Multiply what follows next (0.00014983) with 365 to get 0.05469. Lastly, multiply this figure by 100 to determine what APR percentage you are looking at - 5.45%. When evaluating a loan, you must understand the different types of APRs you may encounter. Purchase APR: The Purchase APR is the regular rate you will charge for a new purchase. Balance Transfer APR: This rate applies to balance transfers. Usually, it is higher than the Purchase APR. Cash Advance APR: This rate applies to cash advances. It is typically higher than both the Purchase and Balance Transfer APRs. Penalty APR: A penalty APR may apply if you are late with a payment or miss payments altogether. It is usually much higher than regular APRs. Introductory/Promotional APR: Some lenders offer promotional APRs as an incentive to open up a new account or take out a loan. These rates may be lower than regular APRs but only last for a limited period. Understanding your APR can help you save money by comparing different loans from various lenders on an equal playing field. Knowing the formula and other APR types is key to wise borrowing decisions. APRs are considered good when they reflect the going market rate plus a modest profit. The benchmark for determining a good APR can swing wildly if riding on the prime rate. This is set by the central bank and reported as a percent for comparison against other potential loans or lines of credit. Some very competitive industries may offer ultra-low or zero introductory APRs, with the caveat that they will skyrocket once the introductory period ends. Also, impeccable credit can qualify for far lower rates than those with more questionable histories, though many providers now offer solutions in both directions. When considering borrowing money, the APR available will vary depending on your credit history. For example, if you have excellent credit, you will likely find that the lowest APR for a credit card could be 12%, while someone with only so-so credit might experience an APR in the high teens. Although these rates can change daily, it is essential to note that those with the highest ratings are usually eligible for some of the best interest rates—around 12% for credit cards and approximately 3.5% for 30-year mortgages. Finding a good APR requires balancing risk against reward, shopping around in one’s demographics to make an educated decision with as little regret as possible. Interest rates and APR are two terms that are often used interchangeably, but they refer to two slightly different concepts. Interest rates and APR are different when taking out a loan. While the interest rate is the annual cost of borrowing expressed as a percentage, APR includes other fees such as mortgage insurance, closing costs, discount points, and origination fees. As such, the APR will be higher than the interest rate. Under federal law, lenders must provide consumers with a Truth in Lending disclosure listing the nominal interest rate and the APR on any consumer credit agreement. This is so that borrowers can compare their loans accurately. All lenders must follow certain rules when computing APR to ensure that the advertised number is accurate. For borrowers with excellent credit in ideal credit conditions, 0% APR deals are available. These deals may come with additional benefits, such as flexible repayment schedules or deferred payments, making them attractive offers for those who qualify. Annual Percentage Rate measures the total cost of a loan, expressed as a yearly rate. It includes interest and any additional fees or charges associated with the loan. Annual Percentage Yield (APY) is also expressed as a yearly rate but takes into account the effect of compounding interest. This means that APY will always be higher than APR as it accounts for the additional interest earned on previously earned interest. APR is the annual rate charged for borrowing or earning money but does not take into account compounding interest. APY, however, does take into account the effect of compounding interest, which can make the total cost of a loan significantly higher than the stated APR. The more frequently the interest compounds, the more significant the difference between APR and APY will be. When making decisions about loans and investments, it is important to understand that while most lenders advertise their rates using APR, investment companies typically provide information on APY instead. The Annual Percentage Rate is an important metric that helps consumers compare the cost of different credit options. It calculates the total cost of borrowing annually, including the interest rate and any other associated fees or charges. Several factors can affect the APR, most notably the borrower's creditworthiness and the length of the lending period. Typical types of APR include introductory and regular rates, fixed or variable rates, and rates that are cash-in-advance or revolve based on periodic billing. Being familiar with these types will help borrowers accurately select viable lending options. Knowing your APR can be invaluable when shopping for loans. This is because it allows you to make meaningful comparisons between loan products with different prices and characteristics to find the best fit for your needs.What Is Annual Percentage Rate (APR)?
How Annual Percentage Rate (APR) Works
Annual Percentage Rate Formula
Types of APRs
What Is a Good APR?
Interest Rate vs APR
Annual Percentage Yield (APY) vs APR
Final Thoughts
Annual Percentage Rate (APR) FAQs
Your credit cards APR can usually be found on your monthly statement or in the terms and conditions of your account.
To calculate APR, you will need to add together the origination fees and total interest paid, divide this result by the principal loan amount, divide again by the length of your loan, multiply the result by 365, and then multiply by 100.
APR takes into account all costs associated with borrowing money, while APY calculates how much interest you will earn if you leave your money invested for a year.
The Fed's current national average APR sits at a competitive 20.40%, which makes it an advantageous rate if your own APR is equal to or below this benchmark.
APR usually refers to the interest charged for a year and is typically not set monthly. However, you may be charged monthly interest if you carry a balance on your credit card. The current APR for your card can be found on your monthly statement or in the terms and conditions of your account.
True Tamplin is a published author, public speaker, CEO of UpDigital, and founder of Finance Strategists.
True is a Certified Educator in Personal Finance (CEPF®), author of The Handy Financial Ratios Guide, a member of the Society for Advancing Business Editing and Writing, contributes to his financial education site, Finance Strategists, and has spoken to various financial communities such as the CFA Institute, as well as university students like his Alma mater, Biola University, where he received a bachelor of science in business and data analytics.
To learn more about True, visit his personal website, view his author profile on Amazon, or check out his speaker profile on the CFA Institute website.
