Conditional Value at Risk (CVaR), also known as Expected Shortfall (ES) or Tail Value at Risk (TVaR), is a risk measure that quantifies the expected loss of an investment or portfolio in the event of extreme market conditions. CVaR is calculated as the average of the losses that exceed the VaR threshold, providing an estimate of the expected loss in the tail of the loss distribution. CVaR is particularly useful for assessing the risk of investments with non-normal return distributions, such as those with fat tails or skewness. It provides a more comprehensive view of tail risk by considering both the likelihood and magnitude of extreme losses. CVaR also plays a crucial role in risk management and portfolio optimization. It helps investors and financial institutions to better understand the potential losses in extreme market conditions, allocate their capital efficiently, and devise effective risk management strategies. While both CVaR and VaR are essential risk management tools, CVaR provides a more detailed view of the tail risk by focusing on the average loss in the worst-case scenarios. This makes CVaR a more conservative and robust measure, which is particularly useful for capturing the risk of assets with non-normal distributions and fat tails. Probability Distribution of Losses: A probability distribution representing the likelihood of different losses associated with an investment or a portfolio. Confidence Level: The level of certainty used in CVaR calculations to define the worst possible outcomes. It is typically expressed as a percentage, such as 95% or 99%. Expected Shortfall (ES): Another term for CVaR, which emphasizes the fact that CVaR calculates the average loss in the worst-case scenarios. Discrete Distribution: In cases where the probability distribution is discrete, CVaR is calculated as the weighted average of the losses beyond the specified confidence level. Continuous Distribution: When the probability distribution is continuous, CVaR is calculated as the integral of the losses multiplied by their corresponding probabilities, beyond the specified confidence level. Numerical Methods (Monte Carlo Simulation): For complex distributions or large portfolios, Monte Carlo simulation can be used to estimate CVaR by simulating a large number of potential market scenarios and calculating the average loss in the worst-case scenarios. Risk Measurement: CVaR provides an estimate of the potential loss in extreme market conditions, which can be used to quantify the risk associated with an investment or a portfolio. Risk Comparison Between Assets or Portfolios: By comparing the CVaR values of different assets or portfolios, investors can make more informed decisions on their investments and risk management strategies. CVaR is widely used in various financial applications, including portfolio optimization, stress testing, risk budgeting, and risk monitoring and reporting. Some of the main applications of CVaR in finance are: CVaR is an essential tool for portfolio optimization, where the goal is to maximize returns while minimizing risk. Portfolio managers use CVaR to identify optimal allocations of assets within a portfolio that can provide the best trade-off between return and risk, taking into account the risk of extreme events. By optimizing the portfolio based on CVaR, investors can reduce their exposure to tail risk and decrease the likelihood of experiencing significant losses during market downturns. CVaR is also used in the pricing and hedging of financial derivatives . It helps to estimate the potential losses associated with the underlying assets or portfolios, which can be used to determine the appropriate price for options, futures, and other derivatives contracts. Additionally, CVaR-based risk measures can be used to hedge portfolios against extreme market events and reduce the likelihood of significant losses. CVaR can be used in asset and liability management (ALM) to assess the risk of mismatches between assets and liabilities under extreme market conditions. This allows institutions to develop strategies to ensure they have adequate liquidity and capital to meet their obligations during adverse market events. Sub-Additivity Property: CVaR satisfies the sub-additivity property, which means that the CVaR of a combined portfolio is always less than or equal to the sum of the individual CVaRs. This property makes CVaR a coherent risk measure, suitable for portfolio optimization and risk management. Focus on Tail Risk: Unlike VaR, which only considers the worst loss at a specific confidence level, CVaR focuses on the average loss in the worst-case scenarios, providing a more comprehensive view of the tail risk. Robustness to Outliers: CVaR is less sensitive to extreme outliers compared to VaR, making it a more robust risk measure for assets with non-normal distributions and fat tails. Computational Complexity: Calculating CVaR can be more computationally demanding than VaR, especially for large portfolios or complex probability distributions. Dependence on Underlying Distribution Assumptions: The accuracy of CVaR estimates relies heavily on the assumptions about the underlying probability distribution of losses, which may not always hold in real-world situations. Lack of Universal Acceptance: While CVaR has gained popularity among academics and risk managers, it has not yet achieved the same level of widespread adoption as VaR in regulatory frameworks and industry practices. Mean-CVaR Optimization: This framework aims to maximize the expected return of a portfolio while minimizing its CVaR, leading to an optimal trade-off between return and risk. Min-CVaR Optimization: In this approach, the objective is to minimize the portfolio's CVaR, subject to certain constraints on portfolio weights and other variables. CVaR-Constrained Optimization: This method involves optimizing the portfolio's expected return, subject to a constraint on its CVaR, ensuring that the portfolio risk remains below a predefined threshold. Linear Programming: CVaR optimization problems can often be formulated as linear programming problems, which can be solved efficiently using standard optimization techniques. Quadratic Programming: Some CVaR optimization problems may require quadratic programming techniques, particularly when dealing with non-linear constraints or objectives. Evolutionary Algorithms (Genetic Algorithms, Particle Swarm Optimization): For complex CVaR optimization problems, evolutionary algorithms such as genetic algorithms or particle swarm optimization can be employed to find near-optimal solutions. CVaR can be used as a key input for stress testing and scenario analysis, allowing financial institutions to assess their resilience to extreme market events and develop contingency plans. CVaR can serve as a basis for risk budgeting and allocation, helping institutions to determine how much risk to take in different asset classes or investment strategies, and allocate capital accordingly. CVaR can be used as a risk monitoring tool, enabling institutions to track their risk exposures over time and report their risk profiles to regulators, investors, and other stakeholders. Basel Committee on Banking Supervision: The Basel Committee has recognized the importance of CVaR and has incorporated it into some of its regulatory frameworks, such as the Fundamental Review of the Trading Book (FRTB). Financial Stability Board: The Financial Stability Board has also acknowledged the usefulness of CVaR in assessing and managing systemic risk in the financial system. National Regulatory Authorities: Some national regulatory authorities have started to adopt CVaR as a supplementary risk measure in their prudential frameworks. Banks and Insurance Companies: Many banks and insurance companies have started to incorporate CVaR into their internal risk management models and capital allocation processes. Hedge Funds and Mutual Funds: Hedge funds and mutual funds have increasingly been using CVaR for portfolio optimization, risk management, and performance evaluation purposes. Pension Funds and Endowments: Pension funds and endowments have also begun to adopt CVaR as a means of assessing and managing their investment risks, as well as optimizing their asset allocations. Conditional Value at Risk (CVaR) has emerged as an essential risk measure in modern finance, providing valuable insights into the tail risk of investments and portfolios. Its robustness, focus on worst-case scenarios, and coherent properties make it an important tool for portfolio optimization, risk management, and regulatory compliance. As the financial landscape continues to evolve, CVaR is expected to gain more prominence in both academia and industry. Ongoing research aims to improve the accuracy and efficiency of CVaR calculations, explore new applications, and further integrate CVaR into risk management and regulatory frameworks.What Is Conditional Value at Risk (CVaR)?
Comparison Between CVaR and Value at Risk (VaR)
Understanding CVaR
Key Concepts and Terminology
Calculation of CVaR
Interpretation of CVaR Results
Applications of CVaR in Finance
Portfolio Optimization
Derivatives Pricing and Hedging
Asset and Liability Management
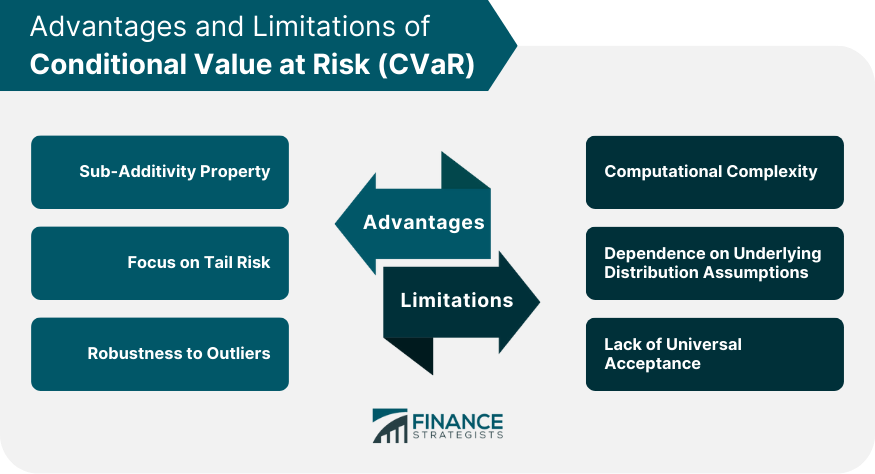
Advantages and Limitations of CVaR
Advantages
Limitations
CVaR in Portfolio Optimization
CVaR-Based Portfolio Optimization Frameworks
Optimization Algorithms
CVaR in Risk Management
Stress Testing and Scenario Analysis
Risk Budgeting and Allocation
Risk Monitoring and Reporting
Regulatory and Industry Perspectives on CVaR
Regulatory Views on CVaR
Adoption of CVaR by Financial Institutions and Asset Managers
Conclusion
Conditional Value at Risk (CVaR) FAQs
Conditional Value at Risk (CVaR) is a risk assessment and management tool used to estimate the potential loss in value of an investment or portfolio under extreme scenarios.
Value at Risk (VaR) measures the potential loss of an investment or portfolio at a specific confidence level, whereas Conditional Value at Risk (CVaR) measures the expected loss beyond that confidence level.
CVaR is typically calculated by taking the average of the worst-case scenarios that fall beyond a specified confidence level. It can be expressed as a dollar value or as a percentage of the investment.
One advantage of using CVaR is that it takes into account the potential losses beyond the confidence level considered by VaR, which can be particularly useful in assessing the risk of tail events. Additionally, CVaR provides a measure of the expected loss, rather than just a single point estimate.
CVaR is commonly used in finance and investment management, where it can be used to assess the risk of individual assets or portfolios. It can also be used in risk management for insurance, supply chain management, and other industries where extreme events can have a significant impact.
True Tamplin is a published author, public speaker, CEO of UpDigital, and founder of Finance Strategists.
True is a Certified Educator in Personal Finance (CEPF®), author of The Handy Financial Ratios Guide, a member of the Society for Advancing Business Editing and Writing, contributes to his financial education site, Finance Strategists, and has spoken to various financial communities such as the CFA Institute, as well as university students like his Alma mater, Biola University, where he received a bachelor of science in business and data analytics.
To learn more about True, visit his personal website or view his author profiles on Amazon, Nasdaq and Forbes.